I'm a little late to this 4%-inflation-target party set off by the economist Lawrence Ball (whose work shows up here at OTE more than random chance would predict) and picked up by Paul K.
I have a few things to add to the discussion, but first, a very brief recap of this simple, sensible argument. Ball contends that when the central bank maintains a 2% target, the economy is over-exposed to zero-lower-bound (zlb) risk when we hit a downturn. Since the real interest rate (r) is the nominal rate (nom) minus inflation (inf), or r=nom-inf, then, as Ball points out, since nom can't go below zero, the real rate can't fall below -inf. In this way, the problem with "...low inflation is that it raises the lower bound on the real interest rate."
That's only a danger if the real rate needs to go below -inf, so one important question here is "how likely is that?" The answer, according to Ball and others, is "more likely than a lot of people think," and there's a lot of analysis showing this to clearly be the case in the great recession which we're still slogging our way out of.
Thus, the danger is not just inflation that's "too low" such that it invokes zlb risk. It's low inflation in tandem with a recession deep enough that we need real interest rates to go well below zero. One lesson to take from this research is that low inflation and asset bubbles are a particularly toxic combination, and they're increasingly common.
That "still slogging" point above is important. Why risk higher inflation just to avoid zlb risk? Because the zlb is a very serious problem that consigns an economy and the people in it to years of slow growth and high unemployment, QED. Ball's suggestion is an insurance policy against that destructive possibility, kind of like suggesting that we put on a few more pounds when the harvests are robust so that when we're forced to eat less in bad times, we'll be able to shed a few more pounds without getting sick.
In this analogy, the 2%'ers are imperious dieticians telling us that we're kidding ourselves if we think we can put on just two more pounds. Once we breach 2%, we'll never stop eating, i.e., inflation won't stop at 4%. But this is far from obvious. If the Fed can anchor inflation at 2%, why can't they do so at 4%?
I've got two things to add, the second more important and interesting than the first.
First, I'd make a somewhat analogous case on the fiscal policy side re the (public) debt/GDP ratio: it's important for the debt/GDP ratio to come down in expansions so that it can go up again in recessions. I clearly don't buy the Reinhart/Rogoff stuff about debt thresholds affecting growth but that doesn't mean we can safely ignore debt ratios. If the debt ratio remains at the same elevated level it is now, as opposed to coming down once a bona fide expansion takes hold, it will be much harder to convince policy makers of the Keynesian logic that when the next downturn hits, the debt ratio needs to go higher still.
In both this and the 4% inflation case, you want these key economic variables perched such that they can effectively respond to recession. Inflation needs to be higher than current targets and the debt ratio needs to be lower at the end of the expansion than at the beginning.
Second, in this and other discussions about why we'd want higher inflation, I've expressed concerns about real wage growth, especially of low-wage workers. They're bearly keeping pace with current very low levels of inflation. Why would anyone who worries about inequality and stagnant earnings advocate a policy that would further lower real wages and incomes?
Because there's a growth tradeoff on offer here. The whole point of the higher inflation rates is to generate more growth and lower unemployment. So the question here is a quantitative one: would the higher inflation generate enough faster wage growth for low- and middle-wage workers to more than offset its impact?
On paper, i.e., based on various standard estimates, the answer is yes. For example, Ball estimates that had the Fed targeted 4% instead of 2% inflation, the unemployment rate would have come down by two more percentage points, 2010-13. According to this figure from EPI which maps unemployment changes onto real wage changes,* a two-point decline in unemployment would raise low wages for men by about 4% and for women by about 3%. Note the much smaller, yet still positive, effects for higher wage workers shown in the figure.
So by these metrics, it's a worthy trade off and I can stop worrying about whether a higher inflation target will result in lower real earnings for lower-wage workers. But how realistic are these links in the chain? They're plausible--mostly back of the envelope but using standard magnitudes.
On the other hand, the figure below might give you pause. It plots annual nominal wage growth for low- and middle-wage men (10 and 50 percentiles) against the advocated 4% inflation target (the figure for women looks similar, though their median does a bit better). Since the mid-1980s, nominal wage growth for these mid- and low-wage men has rarely beat 4%.
At the same time, this is, of course, the period where low-inflation targeting has dominated, so one could argue that this result is kinda baked in the cake.
End of the day, I'd come down with the higher inflation targeters, but I'd keep a very close eye on the real wage trends of the bottom half. Yes, they very much need more growth and lower unemployment. But if it doesn't show up in their real paychecks, it won't help them.
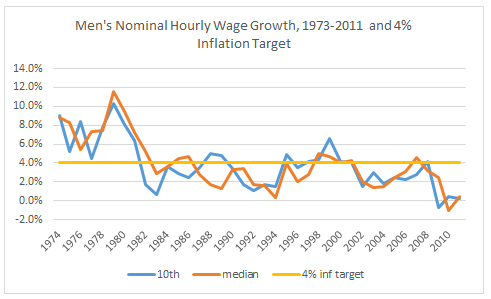
Source: Economic Policy Institute hourly wage data.
*But doesn't the graph say "nominal" wage changes on the y-axis? Yes, but the regression they use to get these elasticities assigns inflation (lagged one period) a coefficient of one--a standard assumption--so that pretty much makes it a regression of real wage changes on unemployment ("pretty much" because of the lag). Another way of making the same point in the text is that if, as assumed in these regressions, for each point of higher inflation, nominal wage growth goes up 1%, then we don't need to worry about the impact of higher inflation on real wage growth.
This post originally appeared at Jared Bernstein's On The Economy blog.
