
Examine an ordinary sandpile created by the continuous pouring of sand, one grain at a time, onto the top of a desk (Figure 1). At first, not much happens. While every now and then you will see small avalanches occurring, the slope of the pile slowly steepens--but only up to a point. Once a certain critical slope is attained, any attempt to add more sand will result in avalanches that will act to preserve that conical shape of maximal slope. With the addition of still more grains, sand will start falling off the desk's edge reaching a state in which sand is lost at the same rate that it is being added.

Figure 1. A sandpile achieves a critical slope.
This type of behavior, in which instead of attempting to reach an equilibrium, a system maintains a global order (in this case, the slope) by means of hovering precariously close to a series of sudden instabilities on all scales (avalanches), has been dubbed "Self-Organized Criticality" (SOC). Physicists Par Bak, Chao Tang, and Kurt Wiesenfeld coined the term in 1987.
Since its introduction, attempts have been made to apply the concepts of SOC to a wide variety of phenomena in which complexity arises, ranging from solar flares and evolutionary biology to neuroscience and economics. What are the key ingredients that make this theory so appealing? These are easy to follow with the simple model of the sandpile. First, the details of the interactions among individual grains of sand are not important, as long as they are describable by a simple set of laws, and the sand is not sticky. More generally, a crucial feature of SOC is the fact that complexity can emerge spontaneously, from local interactions. Second, avalanches can be of any size from that of a single grain to a total collapse. Furthermore, although individual avalanches are unpredictable in size and timing, the frequency of the sizes of avalanches follows what mathematicians refer to as a "negative power law"--catastrophic avalanches are rarer than tiny ones. For instance, at any given period of time you could have (on average) 100 avalanches involving ten grains, ten avalanches involving 100 grains, and only one avalanche involving 1,000 grains. One of the reasons that SOC has been enthusiastically applied, for instance, to earthquakes, is that the Gutenberg-Richter relationship concerning the distribution of earthquake sizes, states that on the average, there is one earthquake of magnitude 8 every year, ten of magnitude 7, about 100 of magnitude 6, and so on. The frequency of aftershocks also exhibits a power-law behavior (known as Omori's law). However, detailed computer models of seismic activity which involve SOC, seem to fail (so far, at least) to produce reliable results, due primarily to the fact that the models do not adequately take into account elasticity in the solid crust.
Astrophysicists are also interested in SOC as potentially being behind a number of phenomena that exhibit interesting statistics. Pulsar glitches, for instance, are tiny, random increases in the rotation rate of radio pulsars. While theories of glitches have mainly focused on the microphysics of the coupling between the superfluid in pulsar interiors and the solid crust, attempts have also been made to describe the global, collective dynamics, by means of SOC. Similarly, a few researchers have suggested that SOC can also describe the overall statistics of solar flares (Figure 2).
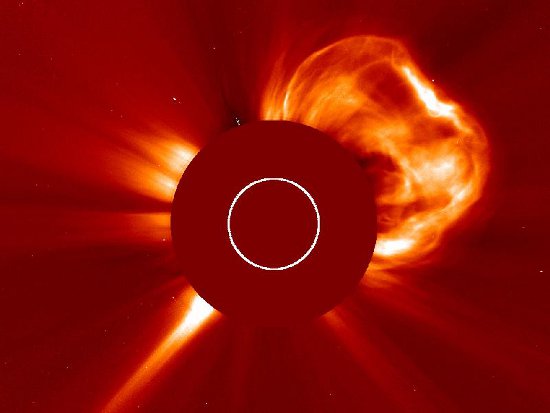
Figure 2. The Solar and Heliospheric Observatory (SOHO) took this image of a coronal mass ejection associated with an X1.8 class solar flare on January 27, 2012 at 1:38 PM EST. It does not initially appear to be Earth-directed, but Earth may get a glancing blow. Credit: SOHO.
An application of SOC that appears to be more promising is to the intricate problem of protein folding. There, experiments have identified many families of proteins that have similar folds. In 2007, researchers Marcelo Moret and Gilney Zebende discovered that hydrophobic interactions responsible for the collapse and folding of proteins can be described in terms of a power law of the solvent-accessible surface area of amino acids (the building blocks of proteins) in small fragments of protein. As I've noted above, a power law behavior is one of the hallmarks of SOC. Another phenomenon that has been successfully analyzed using SOC is that of forest fires. There again, researchers have shown that the system has a tendency to drive itself into a critical state independent of the initial conditions.
Finally, Peter Grassberger, a physicist known for his contribution to chaos theory, suggested an amusing application of SOC to even a bureaucratic environment. Imagine a large office with many bureaucrats sitting with their desks in rows, as in Figure 3. At regular intervals, a piece of paper requiring some action lands on the desk of a random bureaucrat. This person does absolutely nothing with the paper until four (or more) such documents collect on his/her desk. At that point, the bureaucrat sends one paper to each one of his/her four nearest neighbors. All the people in the office behave in like manner, except that those who are near a window simply throw the four papers out the window. You can easily convince yourself that at some point, even a single sheet of paper entering the office will result in many "avalanches."
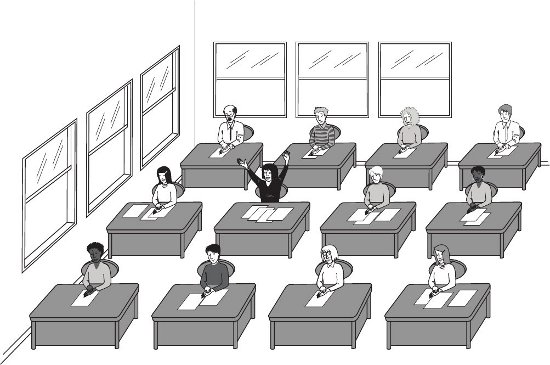
Figure 3. Bureaucracy in action. Every time one finds four or more sheets of paper on his/her desk, he/she sends one to each of his/her four nearest neighbors. Those near a window throw the papers out. Credit: A. Feild.
While not always providing meaningful insights, the amazing thing about self-organized criticality is the fact that we can still learn something profound even from a sandpile!
The few examples I have mentioned here are only the tip of the iceberg. Researchers have examined both natural phenomena, such as mass-accreting black holes and mass extinctions on Earth, and human-driven activities, such as traffic jams and market economics, as potential manifestations of SOC. This could point to an intriguing truth: The critical state may actually be the most efficient state that can dynamically be reached. As Victor Hugo noted in Les Miserables: "How do we know that the creations of worlds are not determined by falling grains of sand?"