In Auguries of Innocence, William Blake beckons the reader to "...hold infinity in the palm of your hand." If we can't see the infinite, how might we touch and explore it? Let's begin by watching the video below, which places this concept into a physical context.
In Toy Story, Buzz Lightyear exclaims, "To infinity and beyond." What could possibly be beyond infinity? In the video above, we see that an infinite amount (such as a rope going off forever in only one direction) can be added to an infinite amount and result in an infinite amount (a rope that goes on forever in both directions). Easy enough, infinity + infinity = infinity.
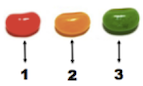
Infinity measures the size of a set like the set of natural numbers N = {1, 2, 3, 4, ...}. Two sets have the same size if you can describe an exact pairing of their elements. In the picture below, our set of jellybeans has the same size as the set {1, 2, 3} since the arrows describe such an exact pairing.
Now, let's visit the Mega-Motel which has infinitely many rooms and is booked solid. A trucker arrives at the front desk and the attendant states, "You'll have room 1." Over the intercom, everyone is asked to move down one room. Does everyone fit? If not, who doesn't? In fact, everyone knows where to go. As such, the sets {0, 1, 2, 3, ...} and {1, 2, 3, ...} have the same size. Notice, the motel can still house 100, 1,000 or 1,000,000,000 travelers. Is the only thing beyond infinity the infinite itself?
Infinity often refers to sets containing numbers of increasing size. In the earlier video, the mime cuts the rope in half, in half again and finally in a manner meant to suggest cutting the rope infinitely many times. There are an infinite amount of real numbers between 0 and 1. Is this set the same size as {1, 2, 3, ...}?
Consider what it means for two sets not to have the same size. Let J be the set of red, orange, green, and yellow jellybeans and T = {1, 2, 3}. These two sets are not the same size since any pairing of the sets will always leave an element of J unpaired with an element in T.
To delve deeper into this concept, let's play Dodge Ball, math style, with two players as described in Coincidences, Chaos, and All That Math Jazz: Making Light of Weighty Ideas by E. Burger and M. Starbird. Player 1 places six O's and X's in the first row of Table 1. Then, Player 2 (the Dodger) puts an X or an O in the first column of Table 2. Player 1 then places six X's and O's in the second row of Table 1. Player 2 chooses an X or an O for the second column of Table 2. After Table 1 and Table 2 are filled, Player 2 wins if the row in Table 2 does not match any row in Table 1. Otherwise, Player 1 wins. Play the game a few times. Notice any optimal strategies?
Table 1
Table 2
If I were the Dodger, I would make the choices for the game seen below.
Table 1
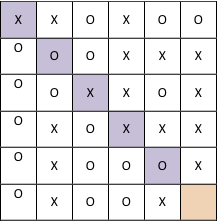
Table 2
For my first column, I look at the first element in row 1 of Table 1 and choose the opposite symbol for my play. For play 2, I look at the second column of the second row of Table 1 and again choose the opposite symbol. If I continue this strategy, Player 1 cannot win -- ever.
In the late 1800's, Georg Cantor analyzed the infinite with this strategy. Suppose the set of real numbers between 0 and 1 are the same size as the set of natural numbers. Then, we can define an exact pairing between all the elements of the sets. Assume this exists. Then, we can't produce a real number between 0 and 1 that isn't paired with an integer in N. However, the Dodger knows how to produce such a number!
Suppose our pairing is
The Dodger will choose the number 0.30330.... Why? Dodger chose the first decimal digit, 3, by looking at the first decimal digit of the number paired with 1 and seeing it is not a 3. The second decimal digit of the number paired with 2 is a 3 so Dodger chose a 0. The third decimal digit of the number paired with 3 is not a 3 so Dodger chose a 3. This pattern would continue for all the digits in the list producing a number that cannot be contained in the pairing.
This is Dodge Ball on an infinite board. Regardless of a pairing between the set of natural numbers and the set of real numbers between 0 and 1, Dodger can create a decimal number not in the pairing. While both sets are infinite, one is more than the other!
Buzz Lightyear was right. We can go to infinity and beyond -- to another sized infinity. What lies beyond that size? There is, indeed, another sized infinity. How? Look it up in a book or on the Internet. Then, work to create a model of the concept that enables you to hold the idea, if only to explore a question.
Mathematics allows us to study abstract ideas in ways that might initially defy intuition. Does more than one size of infinity do that for you? It might. It did for colleagues of Cantor. In fact, Henri Poincaré, a leading mathematician of the day, called Cantor's ideas a "disease." Cantor's work was embraced in time. Accomplished mathematician David Hilbert, who originated the story of an infinite hotel, stated, "No one will drive us from the paradise which Cantor created for us."
Mathematics continually pushes the boundaries of its knowledge... in a way, to infinity and beyond.
Follow Tim Chartier on Twitter: www.twitter.com/timchartier
What if infinite warped space is the overwhelming counter-force -- eventually, initiating a tipping point or intersection by becoming a larger infinity -- more than infinite gravity of singularity?
Anything is possible until proven otherwise. Physical observation describes how but not why. So it's very possible, infinite mass inherently confronted by infinite warped space, a reversal (polar shift like), eventually occurs spurred by a larger more powerful infinite counter force.
We may begin to understand this possibility when we finally recognize the mechanism underlying geomagnetic shift or magnetic pole reversal.
If a larger infinity than singularity exists, implication leads directly to the Big Bang Theory.
An expanding universe complicates this idea, however, theories do change. Expect paradigm shifts in the future.
Another game, demonstrating intersecting and diverging infinities, could make this idea viable.
"If a larger infinity than singularity exists, implication leads directly to the Big Bang Theory."
http://www.youtube.com/watch?v=6Xwl4oVnbhU
"Anything is possible until proven otherwise."
Quite the contrary. In science nothing is truly meaningful until observed. Mathematics, of course, is not science, per se, as it does not deal with nature. It is a completely different intellectual domain, but one that has just as much validity as science, itself. It's actually the only other intellectual domain outside of hard sciences that deserves equal attention.
And yet, elsewhere, you posted comments about Werner von Braun's moral culpability rather than his scientific achievements. I guess I shouldn't pay attention to your philosophical arguments.
Now, I'm going to expand on a comment about Alan Turing I once wrote to you, and you ignored.
While agreeing with you about the disgraceful treatment of Turing, I noted that his own self-destructive behavior caused his downfall. You may not think psychology matters, but I think Turing's is worth examining.
He didn't care what anyone thought about him (except his doting mother; what would Freud say?) He got on well with his colleagues in the lab because they shared his intellectual interests. Other than that, his social interactions consisted only of picking up street hustlers to engage in impersonal homosex.
If he had valued any opinion other than his own, he might have heeded advice, after one of the hustlers stole from him following an argument over the cost of sexual services. Anyone would have explained such is to be expected from rough trade.
Furthermore, anyone would have warned him that going to the police to report the theft would mean confessing to a crime, homosexuality being illegal. No good, and likely disaster, would come from that.
Unfortunately, Turing listened only to himself.
Please don't bother to respond. I wrote this only for the purposes of self-expression and a friend's entertainment.
I don't even know what that means, jf12, besides of a fool speaking about things he doesn't understand.
I'm not sure what ontology has to do with anything, but I'm reminded of the Feynman quote that I posted elsewhere a few days ago!
The Feynmann observation is not valid, in general. Good philosophers are more grounded in reality than great non-applied mathematicians. An ontologically better alternative to set theory is mereology.
There is an infinite number of such axioms which distinguish an equally infinite number of different versions of mathematics. It's basically just a matter of choice.
But anyway, "the set of all different cardinalities" is the part of Cantor's paradise that we are expelled from, no matter what Hilbert said.
:-)